【スマホで読む・わかりやすい】共通テスト数学IIB2022本試【解説・正解・問題】

第２問　正解

ア 1　イ 0　ウ 3　エ 2　オカ,キ -2,2
ク 2　ケ,コ 1, 4 (解答の順序は問わない)
サ,シス b, 2b　セ,ソ 2, 1　タ 2
チツ,テ,ト,ナニ,ヌ -1, 6, 9, 12, 5
ネ,ノ 5,2

〔1〕(1)

$y=f(x)$ のグラフの概形は

$a=0$ のとき

$f(x)=x^3+16$

となる。教科書にある $y=x^3$ のグラフを覚えていれば，それを $y$ 軸方向に水平移動したものであることは想像できる。したがって，①が正しい。

・・・ア

$a<0$ のとき

$f(x)$ を微分すると良い。

$f'(x)=3x^2-6a$

$f'(x)$ はつねに正の値をとるので単調増加であり，極値を持たない。

したがって，$\textsf{\textcircled{0}}$ が正しい。

・・・イ

(2)

$f(x)$ を微分して，増減表を作ると良い。

$f'(x)=3x^2-6a=0$ として
$3x^2=6a$
$x^2=2a$
$x=\pm\sqrt{2a}$

増減表は

$\def\arraystretch{1.5}\begin{array}{|c||c|c|c|c|c|}\hline x&\cdots&-\sqrt{2a}&\cdots&\sqrt{2a}&\cdots\\\hline f'(x)&+&0&-&0&+\\\hline f(x)&\nearrow&&\searrow&&\nearrow\\\hline\end{array}$

$\sqrt{2a}=\sqrt{2}a^{\small{\frac{1}{2}}}$ として

$f(-\sqrt{2}a^{\small{\frac{1}{2}}})$
$=(-\sqrt{2}a^{\small{\frac{1}{2}}})^3-6a(-\sqrt{2}a^{\small{\frac{1}{2}}})+16$
$=-2\sqrt{2}a^{\small{\frac{3}{2}}}+6\sqrt{2}a^{\small{\frac{3}{2}}}+16$
$=4\sqrt{2}a^{\small{\frac{3}{2}}}+16$

$f(\sqrt{2}a^{\small{\frac{1}{2}}})$
$=(\sqrt{2}a^{\small{\frac{1}{2}}})^3-6a\cdot\sqrt{2}a^{\small{\frac{1}{2}}}+16$
$=2\sqrt{2}a^{\small{\frac{3}{2}}}-6\sqrt{2}a^{\small{\frac{3}{2}}}+16$
$=-4\sqrt{2}a^{\small{\frac{3}{2}}}+16$

$p$ の値と実数解の個数の関係は上のようになる。したがって，3 個の共有点を持つのは

$-4\sqrt{2}a^{\small{\frac{3}{2}}}+16<p<4\sqrt{2}a^{\small{\frac{3}{2}}}+16$

のときである。

・・・ウ,エ

次に，$p=-4\sqrt{2}a^{\small{\frac{3}{2}}}+16$ のとき

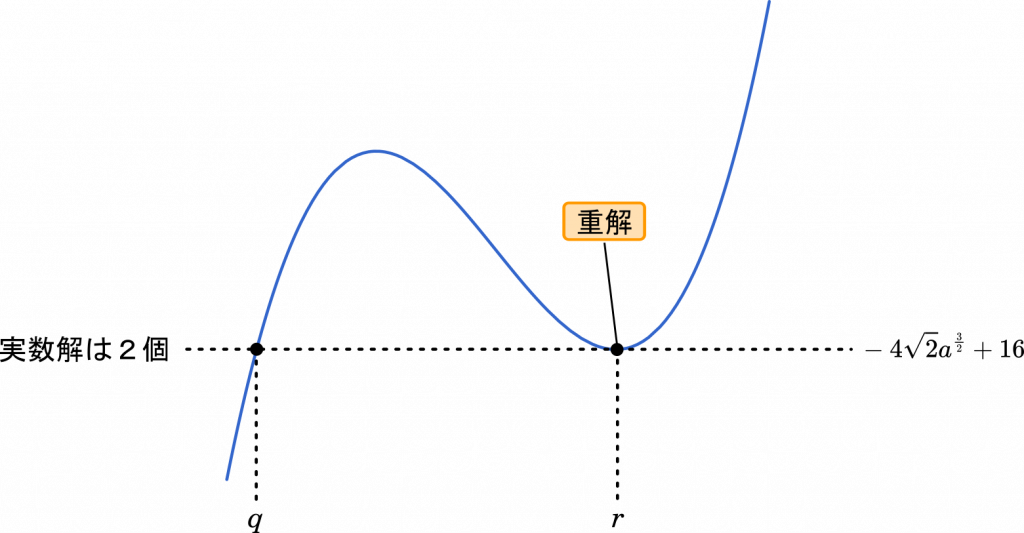
$x^3-6ax+16=-4\sqrt{2}a^{\small{\frac{3}{2}}}+16$ とすると

$x^3-6ax+4\sqrt{2}a^{\small{\frac{3}{2}}}=0$

このとき，この方程式は $x=r=\sqrt{2}a^{\small{\frac{1}{2}}}$ で重解をとる。

よって，方程式は $(x-\sqrt{2}a^{\small{\frac{1}{2}}})^2$ で割り切れる。

組立除法を用いて

$\begin{matrix}1&0&-6a&4\sqrt{2}a^{\small{\frac{3}{2}}}&|\underline{\sqrt{2}a^{\small{\frac{1}{2}}}}\\&\sqrt{2}^{\small{\frac{1}{2}}}&2a&-4\sqrt{2}^{\small{\frac{3}{2}}}\\\hline1&\sqrt{2}^{\small{\frac{1}{2}}}&-4a&0\end{matrix}$

$(x-\sqrt{2}a^{\small{\frac{1}{2}}})(x^2+\sqrt{2}a^{\small{\frac{1}{2}}}x-4a)=0$

$\begin{matrix}1&\sqrt{2}a^{\small{\frac{1}{2}}}&-4a&|\underline{\sqrt{2}a^{\small{\frac{1}{2}}}}\\&\sqrt{2}a^{\small{\frac{1}{2}}}&4a\\\hline1&2\sqrt{2}a^{\small{\frac{1}{2}}}&0\end{matrix}$

$(x-\sqrt{2}a^{\small{\frac{1}{2}}})^2(x+2\sqrt{2}a^{\small{\frac{1}{2}}})=0$

したがって

$q=-2\sqrt{2}a^{\small{\frac{1}{2}}}$，$r=\sqrt{2}a^{\small{\frac{1}{2}}}$

・・・オカ,キ,ク

(3)

(1)より $a<0$ ならば $n=1$

・・・ケ

上の条件を考えれば，②，③は成り立たないことがわかる。

次に，④ と ⑤ を考える。

$a>0$ のとき，実数解の個数は 2 または 3 個であるから，「$a>0$ ならば $n=3$」は成り立たない場合がある。よって，④が正しい。

・・・コ

〔２〕

交点を求めるために式を連立する。ただし，後の解答のため，$g(x)=h(x)$ とせずに，$g(x)-g(x)=0$ としておくと良い。

$g(x)-h(x)=0$
$x^2-3bx+3b^2=0$
$(x-b)(x-2b)=0$
$x=b,2b$

したがって

$\alpha=b,\beta=2b$

・・・サ,シス

次に，$S$ を考える。積分区間は $[\alpha,\beta]=[a,2b]$ である。

このとき，$x^2-3bx+3b^2$ は負の値をとるので，積分の式としては $g(x)-h(x)$ ではなく $h(x)-g(x)$ としなければならない。

$\displaystyle S=\int_\alpha^\beta\{h(x)-g(x)\}dx$

・・・セ

また，$t>\beta$ とすると，積分区間 $[\beta,t]$ では $x^2-3bx+3b^2$ は正の値をとるので

$\displaystyle T=\int_\beta^t\{g(x)-h(x)\}dx$

・・・ソ

よって

$\displaystyle -T=\int_\beta^t\{h(x)-g(x)\}dx$

となるので

$\displaystyle S-T=\int_\alpha^t\{h(x)-g(x)\}dx$

・・・タ

$\displaystyle=\int_\alpha^t -x^2+3bx-2b^2\space dx$

$\alpha=b$ より

$=\Big[-\cfrac{1}{3}x^3+\cfrac{3}{2}bx^2-2b^2x\Big]_b^t$

$=-\cfrac{1}{3}t^3+\cfrac{3}{2}bt^2-2b^2t+\cfrac{1}{3}b^3-\cfrac{3}{2}b^3+2b^3$
$=-\cfrac{1}{3}t^3+\cfrac{3}{2}bt^2-2b^2t+\cfrac{5}{6}b^3$
$=\cfrac{-1}{6}(2t^3-9bt^2+12b^2t-5b^3)$

・・・チツ,テ,ト,ナニ,ヌ

さらに，$S=T$ のとき $S-T=0$ だから

$S-T=0$
$\cfrac{-1}{6}(2t^3-9bt^2+12b^2t-5b^3)=0$
$2t^3-9bt^2+12b^2t-5b^3=0$

$t=b$ とすると

$2b^3-9b^3+12b^3-5b^3=0$

となるので，式は $t-b$ で割り切れる。

組立除法を用いて

$\begin{matrix}2&-9b&12b^2&-5b^3&|\underline{b}\\&2b&-7b^2&5b^3\\\hline 2&-7b&5b^2&0\end{matrix}$

$(t-b)(2t^2-7bt+5b^2)=0$

$2t^2-7bt+5b^2=0$ として

$t=b$ とすると

$2b^2-7b^2+5b^2=0$

となるので，式は $t-b$ で割り切れる。

$\begin{matrix}2&-7b&5b^2&|\underline{b}\\&2b&-5b^2\\\hline2&-5b&0\end{matrix}$

よって

$(t-b)^2(2t-5b)=0$
$t=b,\cfrac{5}{2}b$

$t>\beta$ より $t>2b$ だから

$t=\cfrac{5}{2}b$

・・・ネ,ノ

問題文

〔１〕$a$ を実数とし，$f(x)=x^3-6ax+16$ とおく。

(1) $y=f(x)$ のグラフの概形は

$a=0$ のとき，$\boxed{\boxed{\enspace\textsf{ア}\enspace}}$

$a<0$ のとき，$\boxed{\boxed{\enspace\textsf{イ}\enspace}}$

である。

$\boxed{\boxed{\enspace\text{ア}\enspace}}$，$\boxed{\boxed{\enspace\text{イ}\enspace}}$ については，最も適当なものを，次の $\textsf{\textcircled{0}}$ ～ ⑤ のうちから一つずつ選べ。ただし，同じものを繰り返し選んでもよい。

(2) $a>0$ とし，$p$ を実数とする。座標平面上の曲線 $y=f(x)$ と直線 $y=p$ が 3 個の共有点をもつような $p$ の値の範囲は $\boxed{\boxed{\enspace\textsf{ウ}\enspace}}<p<\boxed{\boxed{\enspace\textsf{エ}\enspace}}$ である。

$p=\boxed{\boxed{\enspace\text{ウ}\enspace}}$ のとき，曲線 $y=f(x)$ と直線 $y=p$ は 2 個の共有点をもつ。それらの $x$ 座標を $q,r(q<r)$ とする。曲線 $y=f(x)$ と直線 $y=p$ が点 $(r,p)$ で接することに注意すると

$q=\boxed{\enspace\textsf{オカ}\enspace}\sqrt{\boxed{\enspace\textsf{キ}\enspace}}\space a^{\small{\frac{1}{2}}}$，$r=\sqrt{\boxed{\enspace\textsf{ク}\enspace}}\space a^{\small{\frac{1}{2}}}$

$\boxed{\boxed{\enspace\text{ウ}\enspace}}<p<\boxed{\boxed{\enspace\text{エ}\enspace}}$ の解答群(同じものを繰り返し選んでもよい｡)

(3) 方程式 $f(x)=0$ の異なる実数解の個数を $n$ とする。次の $\textsf{\textcircled{0}}$ ～ ⑤ のうち，正しいものは$\boxed{\boxed{\enspace\textsf{ケ}\enspace}}$ と $\boxed{\boxed{\enspace\textsf{コ}\enspace}}$ である。

$\boxed{\boxed{\enspace\text{ケ}\enspace}}$，$\boxed{\boxed{\enspace\text{コ}\enspace}}$ の解答群(解答の順序は問わない｡)

〔２〕 $b>0$ とし，$g(x)=x^3-3bx+3b^2$，$h(x)=x^3-x^2+b^2$ とおく。座標平面上の曲線 $y=g(x)$ を $C_1$，曲線 $y=h(x)$ を $C_2$ とする。

$C_1$ と $c_2$ は 2 点て交わる。これらの交点の $x$ 座標をそれぞれ $\alpha,\beta(\alpha<\beta)$ とすると，$\alpha=\boxed{\enspace\textsf{サ}\enspace}$，$\beta=\boxed{\enspace\textsf{シス}\enspace}$ である。

$\alpha\leqq x\leqq\beta$ の範囲で $C_1$ と $C_2$ で囲まれた図形の面積を $S$ とする。また，$t>\beta$ とし，$\beta\leqq x\leqq t$ の範囲で $C_1$ と $C_2$ および直線 $x=t$ で囲まれた図形の面積を $T$ とする。

このとき

$\displaystyle S=\int_\alpha^\beta \boxed{\boxed{\enspace\textsf{セ}\enspace}}\space dx$
$\displaystyle T=\int_\beta^t \boxed{\boxed{\enspace\textsf{ソ}\enspace}}\space dx$
$\displaystyle S-T=\int_\alpha^t \boxed{\boxed{\enspace\textsf{タ}\enspace}}\space dx$

であるので

$S-T=\cfrac{\boxed{\enspace\textsf{チツ}\enspace}}{\boxed{\enspace\textsf{テ}\enspace}}(2t^3-\boxed{\enspace\textsf{ト}\enspace}\space bt^2+\boxed{\enspace\textsf{ナニ}\enspace}\space b^2t-\boxed{\enspace\textsf{ヌ}\enspace}\space b^3)$

が得られる。

したがって，$S=T$ となるのは $T=\cfrac{\boxed{\enspace\textsf{ネ}\enspace}}{\boxed{\enspace\textsf{ノ}\enspace}}\space b$ のときである。

$\boxed{\boxed{\enspace\text{セ}\enspace}}$～$\boxed{\boxed{\enspace\text{タ}\enspace}}$ の解答群(同じものを繰り返し選んでもよい｡)