【数III】e^xとlog xのグラフが接するときの面積を求める／ネイピア数の定義を用いて極限を求める(千葉大2021第8問)

連立方程式をつくる

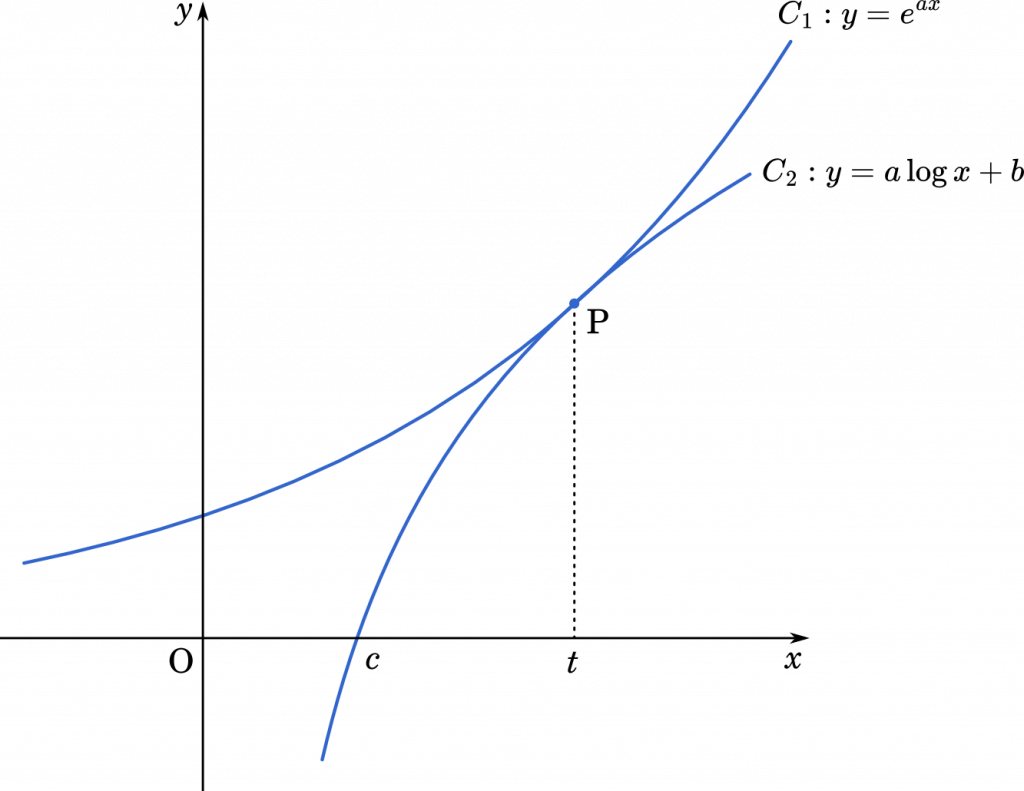
2 つの曲線が $x=t$ で接することから式を作ります。

$e^{ax}=a\log x+b$ ・・・①

また，$x=t$ のとき接線の傾きが一致することからもう一つ式を作ります。

$y=e^{ax}$ より
$y’=e^{ax}\cdot(ax)’$
$=ae^{ax}$

また

$y=a\log x+b$ より

$y’=\cfrac{a}{x}$

$x=t$ のとき，接線の傾きが一致するので

$ae^{at}=\cfrac{a}{t}$
$e^{at}=\cfrac{1}{t}$ ・・・②

両辺の対数をとると

$\log e^{at}=\log\cfrac{1}{t}$
$at\log e=\log1-\log t$

$at=-\log t$
$a=-\cfrac{\log t}{t}$　（答え）

①より $x=t$ とすると

$e^{at}=a\log t+b$

②を代入すると

$\cfrac{1}{t}=a\log t+b$
$=-\cfrac{(\log t)^2}{t}+b$
$b=\cfrac{1}{t}+\cfrac{(\log t)^2}{t}$
$=\cfrac{1+(\log t)^2}{t}$　（答え）

ネイピア数の定義を利用する

まず，$S_1$ の式から作りましょう。

$\displaystyle S_1(t)=\int_0^t e^{ax}\space dx$
$=\cfrac{1}{a}\Big[e^{ax}\Big]_0^t$

$=\cfrac{1}{a}(e^{at}-e^0)$
$=\cfrac{1}{a}(e^{at}-1)$
$=-\cfrac{t}{\log t}(e^{-\log t}-1)$ ・・・③

ここで $e^{-\log t}$ の値を求めます。

$e^{-\log t}=k$ として，両辺の対数をとると

$\log e^{-\log t}=\log k$
$-\log t\log e=\log k$
$-\log t=\log k$
$\log t^{-1}=\log k$
$t^{-1}=k$
$k=\cfrac{1}{t}$

よって，③は

$S_1(t)=-\cfrac{t}{\log t}\Big(\cfrac{1}{t}-1\Big)$
$=-\cfrac{t}{\log t}\cdot\cfrac{1-t}{t}$
$=\cfrac{t-1}{\log t}$

ここから極限を求めます。

$\displaystyle\lim_{t\rightarrow1-0}S_1(t)$
$\displaystyle=\lim_{t\rightarrow1-0}\cfrac{t-1}{\log t}$

とはいえ，このまま極限を求めようにも $\log t$ は $\log1=0$ となってしまいます。そこで，ロピタルの定理を用ると極限は 1 となるので，これを答えとしても構いません。

もし，ロピタルの定理を使わないとしたら少しテクニックが必要です。

まず数IIIの教科書にネイピア数の定義が載っていたことを思い出しましょう。

これを利用していきます。

$\cfrac{t-1}{\log t}=\cfrac{1}{\cfrac{\log t}{t-1}}$

ここで $k=t-1$ とすると $t=1+k$ となります。また，$t\rightarrow1-0$ のとき $k\rightarrow0-0$ となります。

$=\cfrac{1}{\cfrac{1}{k}\cdot\log(1+k)}$
$=\cfrac{1}{\log(1+k)^{\small{\frac{1}{k}}}}$

ネイピア数の定義より

$\displaystyle \lim_{k\rightarrow0}(1+k)^{\small{\frac{1}{k}}}=e$

だから

$\displaystyle\lim_{k\rightarrow0-0}\log(1+k)^{\small{\frac{1}{k}}}=\log e=1$

となります。よって

$\displaystyle=\lim_{t\rightarrow1-0}\cfrac{1}{\cfrac{\log t}{t-1}}=\cfrac{1}{1}=1$　（答え）

さらに log の極限を考える

積分するには，$y=a\log x+b$ と $x$ 軸の交点の座標が必要です。

$a\log x+b=0$ として

$\log x=-\cfrac{b}{a}$

(1)の結果を代入して

$=\cfrac{\cfrac{1+(\log t)^2}{t}}{\cfrac{\log t}{t}}$
$=\cfrac{1}{\log t}+\log t$

このままではどうしようもないので，とりあえず交点の座標を $(s,0)$ として

$\log s=\cfrac{1}{\log t}+\log t$

としておきます。

積分の式をつくりましょう。

$\displaystyle S_2(t)=\int_s^t a\log x+b\space dx$

$\log$ の積分は部分積分を用います。

$\displaystyle=a\int_s^t(x)’\log x\space dx+b\int_s^t dx$
$\displaystyle=a\Big\{\Big[x\log x\Big]_s^t-\int_s^t x\cdot\cfrac{1}{x}\space dx\Big\}+b\Big[x\Big]_s^t$
$\displaystyle=a\Big\{t\log t-s\log s-\Big[x\Big]_s^t\Big\}+b(t-s)$
$\displaystyle=a\{t\log t-s\log s-(t-s)\}+b(t-s)$

もう少し式を整理しておきます。

$=a(t\log t-s\log s)-a(t-s)+b(t-s)$
$=a(t\log t-s\log s)+(b-a)(t-s)$

この状態から極限を考えていきます。

まず

$\displaystyle\lim_{t\rightarrow1-0}a$
$\displaystyle=\lim_{t\rightarrow1-0}-\cfrac{\log t}{t}$
$=0$

これで，$a(t\log t-s\log s)$ の極限は 0 ということになります。

次に

$\displaystyle\lim_{t\rightarrow1-0}b$
$\displaystyle=\lim_{t\rightarrow1-0}\cfrac{1+(\log t)^2}{t}$
$=1$

よって

$\displaystyle\lim_{t\rightarrow1-0}b-a=1$

となります。

さらに $t-s$ の部分の極限を考えます。

$\displaystyle\lim_{t\rightarrow1-0}\log s$
$\displaystyle=\lim_{t\rightarrow1-0}\Big\{\cfrac{1}{\log t}+\log t\Big\}$

ここも，ロピタルの定理を使うと

$\displaystyle=\lim_{t\rightarrow1-0}\Big\{\cfrac{(1)’}{(\log t)’}+\log t\Big\}$

$=0+0=0$

となるので，あっさり答えを出すことができますが，ロピタルの定理を使わない場合も考えてみます。

まず

$\displaystyle\lim_{t\rightarrow1-0}\Big\{\cfrac{1}{\log t}+\log t\Big\}$
$=-\infty+\log 1$
$=-\infty$

となります。

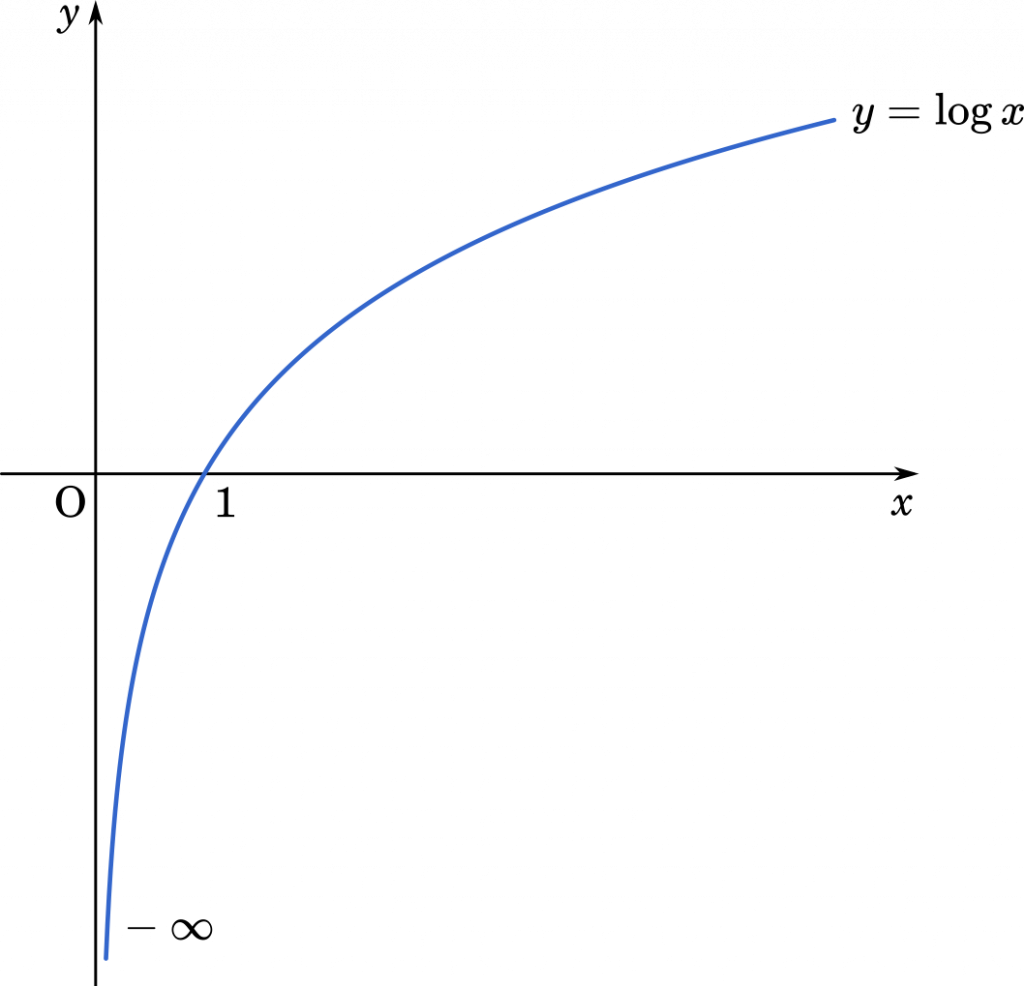
$y=\log x$ のグラフを考えてみましょう。$x$ を 1 より小さいほうから 1 に近づけていくと，$y$ は負の値のほうから 0 に近づいていくことが分かります。

そのため，$\cfrac{1}{\log t}$ は発散するにしても $-\infty$ に発散することに注意しなければなりません。

よって

$\displaystyle\lim_{t\rightarrow1-0}\log s=-\infty$

$\log s$ が $-\infty$ に発散するということは，$s$ は 0 に近づいていくということです。つまり

$\displaystyle\lim_{t\rightarrow1-0}s=0$

が成り立ちます。これより

$\displaystyle\lim_{t\rightarrow1-0}(t-s)=1-0=1$

したがって

$\displaystyle\lim_{t\rightarrow1-0}a(t\log t-s\log s)+(b-a)(t-s)$
$=0+1\cdot1$
$=1$　（答え）